WHT Dome Seeing Investigation |
| Thermal eddies above a telescope structure or mirror which is warmer (or cooler) than ambient, and turbulent mixing of air of different temperatures at the dome aperture will result in wavefront aberrations in addition to the intrinsic seeing. Image spread resulting from such thermal effects within the dome is referred to as dome seeing. We have attempted to quantify any dome seeing at the WHT via analysis of the JOSE data, and comparison of contemporaneous JOSE and DIMM seeing measurements. |
| JOSE - DIMM Seeing comparisons |
| Simultaneous operation of the JOSE and DIMM seeing monitors permits
a direct comparison of the r0 values outside the dome
and at the telescope (GHRIL) focus. The presence of dome seeing would result
in systematically poorer seeing values at the JOSE sensor than for the
DIMM. Substantial periods of simultaneous observations have been
made on a total of 18 nights between 1995 May and 1998 August.
The following figure shows the probability distributions for r0 from the JOSE and DIMM instruments, determined from the contemporaneous data only. No significant difference between the distributions is apparent, demonstrating that the JOSE r0 values were not significantly affected by dome seeing. |
| Normalised probability distributions for contemporaneous JOSE (solid line) and DIMM (broken line) seeing measurements, determined from 2998 JOSE measurements and 4500 DIMM measurements on 18 nights between 1995 May and 1998 August. |
| The figures below show examples of contemporaneous JOSE and DIMM seeing (r0) estimates for three different nights. Individual seeing values for both JOSE and DIMM were each derived from continuous image motion data of 30 seconds duration. Observations were of bright stars at less than 30 degrees zenith distance, and zenith distance corrections were applied for both JOSE and DIMM. |
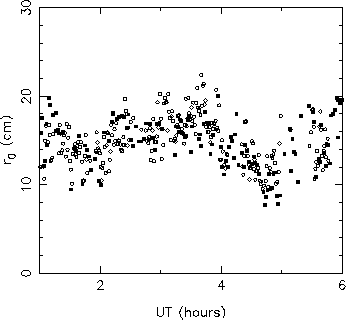
August 16, 1997.
|
Examples of contemporaneous JOSE (filled squares) and DIMM (open circles) seeing measurements
| Since the DIMM tower and WHT are separated by approximately 100 metres, and the JOSE and DIMM sensors did not necessarily observe the same star at the same time, we do not expect perfect correlation of the seeing values on timescales of a few seconds. The instruments observed through different `patches' of atmospheric turbulence at any given time, and so were subject to different random fluctuations of the seeing on short timescales. However no systematic differences between the JOSE and DIMM values are apparent, and seeing changes over timescales of several minutes to hours are well correlated. |
| JOSE Structure Function Measurements |
| Kolmogorov's theory applies to fully developed turbulence in the atmosphere,
and is unlikely to be a good description of the turbulence above a warm
mirror or at the dome aperture. Hence we do not expect a Tatarski structure
function for dome and mirror seeing. The spatial spectrum is expected to
be flatter, with more power at small scales relative to the Kolmogorov
model. For example, Bridgland and Jenkins(1997),
predict a 2/3 power law for the phase structure function due to turbulence
above a warm mirror.
Noll (1976) calculated the expected variances of the Zernike mode coefficients for the Tatarski phase structure function. For this model, low order modes carry more phase variance than higher orders, and the variances are each proportional to r0-5/3 . If strong dome seeing were present we would expect to observe a significant departure from the Noll distribution of mode variances. The following figure shows the mean distribution of mode strengths
for the JOSE data. The rms. values for each data sample have been normalised
to an effective r0 value of 20cm by dividing each term by (r0/0.2)5/3,
and averaged over the whole JOSE data set. Clearly the average distribution
of mode strengths is very close to that predicted by Noll, suggesting that
dome seeing does not in general make a large contribution to the phase
structure function for the spatial scales sampled by the JOSE sensor.
|
| Mean values of the rms. Zernike coefficient values for the whole JOSE data set, normalised to r0 = 20cm and plotted versus the theoretical (Noll) values for r0 = 20 cm. Values of n indicate Zernike radial order. This plot illustrates that the mean relative distribution of mode strengths is close to that predicted by Noll for free-atmospheric seeing. |
| Spot widths |
| Wavefront distortions on scales smaller than the 50cm subaperture diameter
of the wavefront sensor cannot be measured directly from the JOSE data,
since they do not contribute significantly to the individual spot motions.
Hence if there were a contribution from mirror or dome seeing which existed
only on small spatial scales (i.e.. with an outer scale length of 50cm
or less), it would be overlooked in the standard JOSE seeing analysis.
However we would expect such small scale aberrations to increase the FWHM of long exposure images from the sub-apertures of the JOSE sensor. Hence by comparing measured spot FWHM to their values predicted from the JOSE r0 estimates , we can determine whether there is any excess image spread due to unmeasured seeing aberrations. The figure below shows this analysis for the JOSE data set. Measured FWHM are plotted against the predicted spot widths for each data sample. The long exposure image was obtained by summing the exposures in each data sample. Spot FWHM for all unvignetted subapertures were found by fitting Gaussian profiles of variable width, binned to match the JOSE CCD pixel size. |
| Measured versus predicted long exposure widths of
the JOSE subaperture images, for the entire JOSE data set.
The predicted spot width was given by 0.98 lambda/r0 , for the value of r0 measured using the Zernike variance analysis for the data set. In good seeing the true theoretical FWHM is slightly over-estimated by this value, since the subaperture diameter is not much greater than the value of r0 at the observing wavelength, centred at 700nm. Hence we have corrected the theoretical FWHM using values obtained from an analytic derivation for the seeing limited PSF . In this figure, a fixed amount has been added in quadrature to the predicted FWHM, so as to minimise the mean square difference between the predicted and theoretical FWHM over the whole JOSE data set. This amount, 0.53 arcseconds, is then the mean image spread due to all aberrations other than the free atmosphere seeing. With this factor included, we see that the measured and predicted values are well correlated. The rms difference between the measured and predicted widths is 0.06 arcseconds. This amount of scatter is expected from the combined uncertainties in the estimation of r0 and the spot FWHM. The empirically determined FWHM of the PSF for individual subapertures of the JOSE instrument itself is 0.45 arcseconds. We also expect a contribution from small scale figure errors of the WHT mirrors of approximately 0.25 arcseconds added in quadrature to this value, yielding a FWHM of 0.5 arcseconds for the combined telescope and instrument PSF in the absence of seeing. Since the JOSE observations are made without the use of an autoguider,
some drift in the telescope pointing is also expected to add to the long
exposure PSF width. The expected tracking error during a typical JOSE of
one minute duration is roughly 0.05 arcsec. This easily accounts for the
remaining discrepancy between the measured and predicted spot widths. Hence
we conclude that there is no significant contribution from undetected local
seeing on small spatial scales.
|