 ) will undergo peak shifts of aproximately one half of
that which would be predicted for a collimated beam at the maximum angle
of incidence of the cone.
) will undergo peak shifts of aproximately one half of
that which would be predicted for a collimated beam at the maximum angle
of incidence of the cone.




Utilising the filter in uncollimated (i.e. convergent or divergent) light involves slightly more complex considerations. Here, light enters the filter at a range of angles, so that different rays undergo unequal wavelength shifts. This results in not only a central wavelength shift, but a broadening of the bandwidth and lower peak transmission.
As a rough approximation, relatively uniform beams (with full cone angles
less than 20 ) will undergo peak shifts of aproximately one half of
that which would be predicted for a collimated beam at the maximum angle
of incidence of the cone.
) will undergo peak shifts of aproximately one half of
that which would be predicted for a collimated beam at the maximum angle
of incidence of the cone.
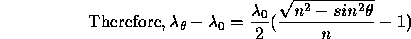
The filters were specified for a focal ratio of 4.5, having a maximum angle
of incidence of the cone of 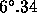 , and a refractive index of the dielectric stack of 2.1.
, and a refractive index of the dielectric stack of 2.1.
Substituting these values in the equation,
lambda_theta - lambda_0 = -0.00069lambda_0
The focal ratio of the INT prime focus is 3.29, which gives a maximum angle
of incidence of the cone of 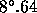 , so, substituting again into
the equation,
, so, substituting again into
the equation,

Therefore, at the INT prime focus, the central wavelength (i.e. the wavelength
of peak transmission) is shifted towards shorter wavelengths by 0.06% from
the specified central wavelengths, or 0.13% from the
measured central wavelength in a collimated beam.
For example, for an 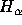 filter
with specified central wavelength 6558Å, the effective central wavelength
will be 6549.6Å.
filter
with specified central wavelength 6558Å, the effective central wavelength
will be 6549.6Å.
Similarly for the WHT prime focus the maximum cone angle is
 ,
and the central wavelength is shifted towards shorter wavelengths by
0.10% from the specified central wavelength, or 0.17% from the measured
central wavelength in a collimated beam.
,
and the central wavelength is shifted towards shorter wavelengths by
0.10% from the specified central wavelength, or 0.17% from the measured
central wavelength in a collimated beam.